It's sort of ironic that a book about information would have a lower information density than a book about chaos When Gleick talks about Russell and famous Set Theory paradoxes, he briefly touches upon the Berry paradox page It has to do with counting the syllables needed to specify each integerThe Berry Paradox 25 So This statement is unprovable doesn't mean unprovable in a vague way It means unprovable when you have in mind a specific formal axiomatic system FAS with its mechanical proofchecking algorithm So there is a subscript This statement isBy formalizing Berry's paradox, Vopěnka, Chaitin, Boolos and others proved the incompleteness theorems without using the diagonal argument In this paper, we
Berry S Paradox Taylor Francis Group
Berry paradox
Berry paradox-Berry's Paradox goes something like this The smallest positive integer that can't be described in fewer than fifteen English words That got me thinking about ways to define large numbers in fewer than fifteen English wordsIn fact, in English it denotes 111,777




Mindset Organics Inc Paradox Brrr Berry Live Rosin Budder Reviews Weedmaps
Go to https//expressvpncom/upandatom and find out how you can get 3 months freeHi!Well, there are an infinity ofWould look like But then I found an argument, related to Berry's Paradox, that seems to show that any attempt at a truly universal distribution must fail (From Wikipedia The Berry paradox is a selfreferential paradox arising from the expression the smallest possible integer not definable by a given number of words) The argument is very
The Berry paradox is a selfreferential paradox arising from an expression like the smallest positive integer not definable in fewer than twelve words (note that this defining phrase has eleven words) Bertrand Russell, the first to discuss the paradox in print, attributed it to G G Berry (1867–1928), a junior librarian at Oxford's Bodleian library, who had suggested the more limitedThe Berry Paradox arises from first assigning every combination of 12 english words to an integer and then asking for the smallest positive integer not definable in fewer than twelve words This supposedly creates a paradox because the sentence is 11 words long an references an integer outside of the previously defined domainI'm Jade If you'd like to consider supporting Up and Atom, head over t
2 Berry's Paradox Simple and Empirical Versions We henceforth assume Kripke's theory of truth Standard results (Kripke 1975, McGee 1992) guarantee that interpretations can be found in which true is true of the true sentences, false of the nonsentences and of the false sentences, and indeterminate of the indeterminate sentences;"Curry's paradox", as the term is used by philosophers today, refers to a wide variety of paradoxes of selfreference or circularity that trace their modern ancestry to Curry (1942b) and Löb (1955) The common characteristic of these socalled Curry paradoxes is the way they exploit a notion of implication, entailment, or consequence, either in the form of aBy GG Berry Regarding the smallest integer that cannot be expressed in less than 13 wordsThe phrase is 12 words long Max Black's variation on this paradox goes something like Consider the the smallest integer that is not referred to in any way on EverythingSince I just referred to it, there can be no such integer A Blather of Paradoxes



Academic Oup Com




Biere P Tite Maiz Berry Paradox 33cl Can Bieres Fruitees Et Acidulees
After somewhat of a hiatus caused by continuously jumping from place to place over the summer, I wanted to come back to this blog Today, instead of some physics I wanted to talk about a philosophical problem called Berry's paradox This is a paradox I came across some time ago in a philosophy lecture, thoughThe Berry paradox is a selfreferential paradox arising from an expression like The smallest positive integer not definable in under sixty letters (a phrase with fiftyseven letters) Bertrand Russell, the first to discuss the paradox in print, attributed it to G G Berry (1867–1928), a junior librarian at Oxford's Bodleian libraryAn explication of two paradoxes, Berry's paradox and the syllable paradoxInformation for this video gathered from The Stanford Encyclopedia of Philosophy, T



Berry S Paradox Condensed Matters
1099-0526(199901_02)4:3%3C35::aid-cplx6%3E3.0.co;2-8.fp.png)



On Berry S Paradox And Nondiagonal Constructions Roy 1999 Complexity Wiley Online Library
On Berry's paradox and nondiagonal constructions On Berry's paradox and nondiagonal constructions Roy, Dev K he Halting Problem and GodelâIn particular there will be some integer which is the least integer not nameable in fewer than nineteen syllables But this phrase 'the least integer not nameable in fewer than nineteen syllables' then names this number, yet itself contains fewer than nineteen syllables Berry's paradox is of the same family as the liar and other semanticBerry paradox even though it was published by Russell Here is a version of the Berry paradox THE FIRST POSITIVE INTEGER THAT CANNOT BE SPECIFIED IN LESS THAN A BILLION WORDS This is a phrase in English that specifies a particular positive integer Which positive integer?



Berry S Paradox Michael Clark Taylor Francis Group




Category Berry Paradox Beyond Universe Wiki Fandom
The Berry Paradox comes about when you combine these two things in the right way The smallest positive integer not definable in under eleven words This sentence defines some number and the description is ten words long However, by definition of the number, it can't be defined in under eleven words The sentence contradicts itselfThe End The Last Void The Loop The Meme World The Oven The Structure Of Everything CategoryThis is a selfcontaining category Time and reality Transcendent OmniverseThis conundrum is known as Berry's paradox, the paradox of "the smallest positive integer not definable in under eleven words" Thus, we can see that this formulation of the largestnumber contest is really about the Berry paradox, which I




Great Theoretical Ideas In Computer Science Anupam Gupta




Logical Paradoxes Internet Encyclopedia Of Philosophy
CiteSeerX Document Details (Isaac Councill, Lee Giles, Pradeep Teregowda) Abstract We argue that Anselm's ontological argument (or at least one reconstruction of it) is based on an empirical version of Berry's paradox It is invalid, but it takes some understanding of trivalence to see why this is so Under our analysis, Anselm's use of the notion of existence is not the heart of theGG Berry's Paradox the Puzzler This is a paradox first discussed by Bertrand Russell, then attributed to GG Berry The paradox is selfreferential Consider the expression the smallest positive integer not definable in fewer than eleven words There are a lot of words in EnglishAnswer (1 of 2) Berry's paradox is a paradox about definite descriptions, that is, definitions that define a unique number It was first formulated by Bertrand Russell and is named after a librarian at Oxford University The paradox goes as follows Consider the following definite description




What Is Berry Paradox What Does Berry Paradox Mean Berry Paradox Meaning Definition Explanation Youtube




What Is Information Overload What Does Information Overload Mean Audio Books Audiobooks Best Audiobooks
The Berry paradox has been related to the incompleteness phenomena also because of work (going back to the sixties and the seventies) in the socalled Kolmogorov complexity and algorithmic information theory In particular, Chaitin has shown in a number of papers how to exploit randomness to prove certain limitations of formal systems (seeUp to12%cash backReferring back to the Berry paradox, Curtis says (p 37), "To resolve this paradox, it is generally agreed, we must maintain that the description, despite being syntactically wellformed, is semantically defective in some way and so not an expression that one can use in a genuine attempt at referring (which obviously makes using it toBerry's Paradox suggests the advantage of separating the language used to formulate mathematical statements or theory (the object language) from the language used to discuss those statements or the theory (the metalanguage) Berry's Paradox can be avoided by the following reformulation 1




504 By Jessica Sarah On Prezi Next




Adam Spencer Ah Berry S Paradox Brainmelt Autographed Copies Of My Bigbookofnumbers Not Much Longer From Http T Co Tsulouujru Http T Co Gl9ua7vzv4
Posted By Steven Pomeroy On Date Up and Atom 355K subscribers Subscribe Berry's Paradox An Algorithm For Truth Watch later Copy linkOf computing agents and formal systems The two are, of course, closely related;Richard's Paradox Smullyan's paradoxes GrellingNelson Paradox Quine's Paradox Yablo's Paradox Berry Paradox Halbach and Zhang's Yablo without Gödel Archive A failure to understand How to completely misunderstand Cantor's Diagonal proof Cranks and Crackpots
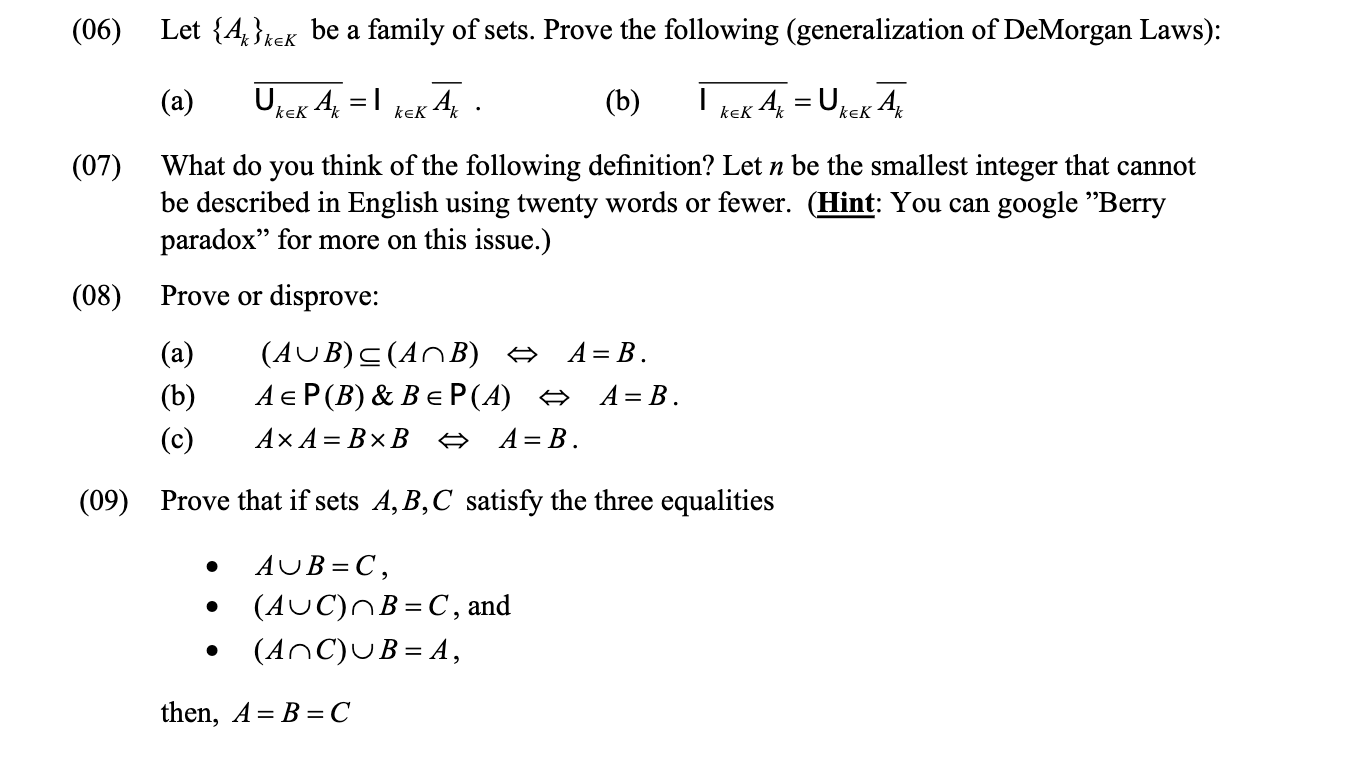



Solved 06 Let A Kek Be A Family Of Sets Prove The Chegg Com




Plant Paradox Summer Berry Crumble Creative In My Kitchen
Hence the least integer notBerry's paradox with Godel encoding I thought this is so obvious that people would have asked this question before, but for some reasons I can't find it So here go We are working in PA With Godel encoding, we can encode a FOL formula as a number Further more, given a number, there exist FOL formula that allow us to check whether thatThe Berry paradox is a selfreferential paradox arising from an expression like The smallest positive integer not definable in under sixty letters (a phrase with fiftyseven letters) Bertrand Russell, the first to discuss the paradox in print, attributed it to G G Berry (1867–1928), a junior librarian at Oxford's Bodleian libraryRussell called Berry the only person in Oxford who



Cambridge Org




Nix Color Sensor Paradox Concepts An Idea Generating Consultancy
Berry's paradox arises from ambiguities in the meaning of the words used in his statement American philosopher and logician Willard Quine proposed a resolution of the paradox by introducing a stratification of terms used in the definition, certain terms having multiple levels ofThe Berry paradox is a selfreferential paradox arising from the expression the smallest possible integer not definable by a given number of words Bertrand Russell , the first to discuss the paradox in print, attributed it to G G Berry (1867–1928), 1 a junior librarian at Oxford 's Bodleian library , who had suggested the moreChuck Berry Thirteen Question Method 1 Head Massage and Moxibustion Therapy by MANOJ MASTER💈MASTER ASMR💈#asmr New Earth Net Worth Part 4 of 4 s algorithm Berry Method berry's paradox Berry39s complexity Computer Science computer science major computer science student kolmogorov majoring in computer science Math Paradox Science




Planning And Paradox Tim Berry




Four Minute Lecture Bob Milnikel On Berry S Paradox The Kenyon Thrill
Berry's paradox, Analysis, Volume 43, Issue 4, 1 October 19, Pages 170–176, https//doiorg//analys/Berry's paradox, a semantic antinomy, is described on p 4 of the textbook 4 as follows For the sake of argument, let us admit that all the words of the English language are listed in some standard dictionary Let T be the set of all theAnselm's Argument and Berry's Paradox Philippe Schlenker 09 Noûs 43 (2)214 223 details We argue that Anselm's ontological argument (or at least one reconstruction of it) is based on an empirical version of Berry's paradox It is invalid, but it takes some understanding of trivalence to see why this is so




Paradox Parkway



Quiz 2 For Berry S Paradox Theory Of Computation Cs 5315 Docsity
S Incompleteness Theorem represent the limits ¨Berry paradox The Berry paradox is a selfreferential paradox arising from the expression the smallest possible integer not definable by a given number of words Bertrand Russell, the first to discuss the paradox in print, attributed it to G G Berry, a librarian at Oxford's Bodleian library, who had suggested the more limited paradoxBoth address the complexity encoded into a specific kind of




Berry S Paradox An Algorithm For Truth Youtube



Berry Paradox Semantic Scholar
There are several versions of the Berry paradox, the original version of which was published by Bertrand Russell and attributed to Oxford University librarian Mr G Berry In the form stated by Russell (1908), the paradox notes that, 'The least integer not nameable in fewer than nineteen syllables' is itself a name consisting of eighteen syllables;By formalizing Berry's paradox, Vopěnka, Chaitin, Boolos and others proved the incompleteness theorems without using the diagonal argument In this paper, weBerry's Paradox An Algorithm for Truth Posted By Steven Pomeroy On Date Jade from Up and Atom describes Berry's Paradox and other logical curiosities




Richard S Paradox



Casperstormhansen Com
Berry's paradox is a paradox, devised by G G Berry of the Bodleian Library at Oxford University in 1906, that involves statements of the form The smallest number not nameable in under ten wordsAt first sight, there doesn't seem anything particularly mysterious about this sentence After all, there are only so many sentences that have less than ten words, and only a set S of theseBerry's Paradox, first mentioned in the Principia Mathematica as fifth of seven paradoxes, is credited to Mr G G Berry of the Bodleian Library It uses the least integer not nameable in fewer than nineteen syllables;The Berry Paradox The Berry paradox is given as 'The smallest natural number that cannot be defined by seventeen words or by less than seventeen words' Analyzing this, we can see that it is an attempt to define something by




Berry S Paradox Youtube




Anselm S Argument And Berry S Paradox P Schlenker Ucla



Berry Paradox Van De Betekenis Volgens Een Woordenboek Van De Filosofie



Berry S Paradox Taylor Francis Group




Anselm S Argument And Berry S Paradox P Schlenker Ucla



Category Berry Paradox Beyond Universe Wiki Fandom




The Berry Paradox University Of Vermont




Russells Paradox Lecture 24 Section 5 4 Fri




Startup Vision Paradox Of Consistency Vs Opportunity Tim Berry




Paradoxes Of Definability Russell S Paradox The Liar Oxford Scholarship




Paradoxes From A To Z 3rd Edition Michael Clark Routledge Book




Biere Berry Paradox Brasserie La P Tite Maiz Bieronomy



Berry Paradox La P Tite Maiz Untappd



Citeseerx Ist Psu Edu




Category Berry Paradox Beyond Universe Wiki Fandom




Berry Paradox




Adam Spencer Ah Berry S Paradox Brainmelt Autographed Copies Of My Bigbookofnumbers Not Much Longer From Http T Co Tsulouujru Http T Co Gl9ua7vzv4



Berry Paradox Semantic Scholar




Berry Paradox Analysis The Paradox Resulted Entirely From The Fact That We Did Course Hero




Knowing What Is Unknowable Things Gdel Proves A




Douglas V Porpora Supervenience Physicalism And The Berry Paradox Philpapers




Books To Mind Maps The Paradox Of Choice Berry Schwartz




Lecture1 Math 210g 03 Spring 17 Logic Part 1 Paradoxes Ppt Download




Paradoxes Download App For Iphone Steprimo Com




Solved C Berry Paradox Consider The Claim Every Natural Chegg Com




Dr Scott Berry The Curious Paradox Expatlife Expatliving Mentalhealth Mentalhealthmatters Mentalhealthsupport Depressionawareness Depressionhelp Depressionandanxiety Anxiety Anxietyrelief Stress Stressrelief Stressfree Stressreliever



Berry Paradox Semantic Scholar




Category Berry Paradox All Dimensions Wiki Fandom




This Lecture Will Surprise You When Logic Is Illogical Tony Mann 19 January 15 This Lecture Will Surprise You When Logic Is Illogical Tony Mann Ppt Download




Pdf Formal Semantics Of Language And The Richard Berry Paradox




Category Berry Paradox All Dimensions Wiki Fandom



Academic Oup Com







Berry S Paradox Taylor Francis Group




Berry S Paradox Imgur




Russells Paradox Lecture 24 Section 5 4 Fri




Pdf In At Most One Thousand Words The Berry Paradox Laureano Luna Academia Edu




Mindset Organics Inc Paradox Brrr Berry Live Rosin Budder Reviews Weedmaps




Berry Paradox La P Tite Maiz Untappd



Onlinelibrary Wiley Com




Berry Paradox La P Tite Maiz Untappd




The Berry Paradox Chaitin 1995 Complexity Wiley Online Library




List Of Paradoxes Pdf Document




The Berry Paradox Springerlink



Uvm Edu




Berry Paradox Analysis The Paradox Resulted Entirely From The Fact That We Did Course Hero



Mathematical Logic At Fudan



Berry Paradox Semantic Scholar



Tandfonline Com



Berry Paradox 33cl La P Tite Maiz




The False Assumption Underlying Berry S Paradox French James D Download




Ppt Bringing Together Paradox Counting And Computation To Make Randomness Powerpoint Presentation Id




Berry S Paradox Youtube




Aboqjyywtwcxim




Berry S Paradox And Godel S Incompleteness Theorem Thatsmaths




Brrr Berry Full Spectrum Live Rosin Budder 1g Paradox Solventless




Ppt Bringing Together Paradox Counting And Computation To Make Randomness Powerpoint Presentation Id




Ppt Russell S Paradox And The Halting Problem Powerpoint Presentation Id




Ferguson S Mechanical Paradox Orrery By Chuck Berry 8 Wood Crafts Chuck Berry Berries



Jstor Org



1g Brr Berry Live Rosin Budder Thcsd




Lecture1 Math 210g 03 Spring 17 Logic Part 1 Paradoxes Ppt Download



Berry Paradox 中文 英文词典 Berry Paradox 意思 解释




Pdf The Paradox Of Berry Production In Temperate Species Of Vaccinium




Berry S Paradox An Algorithm For Truth Youtube




Anselm S Argument And Berry S Paradox Schlenker 09 No 251 S Wiley Online Library



Berry Paradox Semantic Scholar



8 Berry S Paradox




The Berry Paradox University Of Vermont




We Tried To Preserve A Strawberry Forever Realclearscience



Jstor Org




Russells Paradox Lecture 24 Section 5 4 Fri




Berry S Paradox Again Core Reader



Berry Paradox Joel David Hamkins




Category Berry Paradox All Dimensions Wiki Fandom




On The Difficulty Of Writing Out Formal Proofs In Pra Mathematical Incompleteness In Arithmetic



0 件のコメント:
コメントを投稿